jwilzewske: The Physical Properties of Sound Itself: Whitacre & Choral Dissonance

A ural relationships at times bear little relationship to harmonic analysis, or even harmonic function. Stated another way, singing in tune is the result of complex aural/acoustic relationships between and among tones. Those relationships assume a life all their own when placed within the context of vertical harmonic structures. While those relationships appear to defy traditional theoretical knowledge—especially with respect to chromatic movement within harmonic structures—it is clear that choral intonation is a function of the relationship of the tone sung to the harmonic context at that moment. It must be realized that we are using the solfege syllables of Guido d’Arezzo as an intonational device—just as the violinist uses the fingers to ‘place’ a note correctly within its proper intonational context.”
— James Jordan, preface to Matthew LaPine, Prognosticating the ensemble’s ear. in ‘Evoking Sound: The Choral Rehearsal,’ GIA, 2007. (Ch. 16, pp. 147-55).

L ux,
calida gravisque pura velut aurum.
et canunt angeli moliter
modo natum.

Light,
warm and heavy as pure gold.
And the angels sing softly
to the new-born babe.”
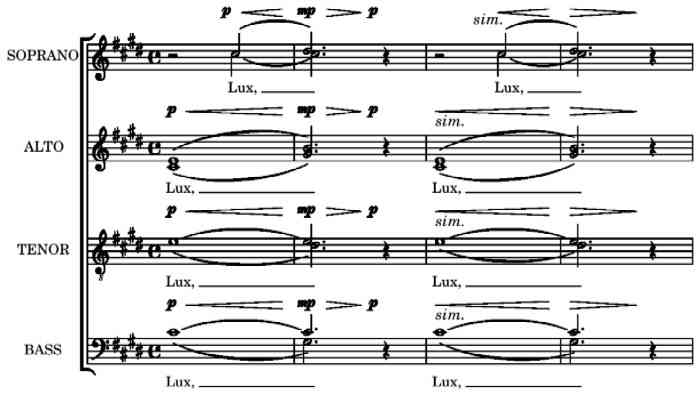
— Edward Esch (original poem in English), Charles Anthony Silvestri (Latin translation).

H ow exactly does Eric Whitacre’s ‘Lux Aurumque’ work? How can it move us so deeply, so mysteriously? It does this in part through Whitacre’s extensive, deliberate use of beat-frequencies between the parts. For me—and maybe for you as well—the effect is not just the richness of a clashing pair of notes; it’s also the ‘phantom’ note that the beat-frequency of a note-pair implies: the ‘implied’ note that we hear when the dissonance is sounded. That implied or ‘phantom’ note has a pitch equal to the frequency difference between the two dissonant notes—the beat-frequency of the difference in frequency. Close-harmony like this is like a human analogue of ‘voix celeste’ on an organ—phantom notes expressly created by the dissonance beat-frequency. Yes, a choral tune is set as a cantus firmus—but it isn’t purely horizontal: there are all of the vertical/harmonic implications as well as the contrapuntal associations...

Superposition of sine waves of two frequencies

M athematically, we should hear the sine wave (f₁ + f₂)/2 as the average of the two pitches. But the sine part of the right side function alternates between negative and positive values many times during one period of the cosine part, and when this happens only the absolute value of the envelope is perceived. So the beat-frequency we hear is just the difference between the two frequencies: (f₁ − f₂).

T he violinist Giuseppe Tartini was the first to document this musical effect in the 18^th Century, calling it il Terzo Suono (Italian for ‘third sound’). Playing pure harmonies (say, a frequency pair whose members have a simple proportional relation to each other, like 4/5 or 5/6, as in the ‘just intonation’ major and minor third respectively) on the two upper strings, such as the C5 against an open E-string E5, gives the ‘phantom’ C3 two octaves lower.

T he major second (M2) interval of Soprano 2 singing C#5 against Soprano 1 singing D#5 in mm. 2, 4, etc. of ‘Lux Aurumque’ gives the implied C#2 three octaves lower.

T he minor second (m2) between Tenors singing D#4 and E4 in those same bars gives pulsations too low to hear as a pitch per se. This is because, when the dissonance involves two pitches that are close together and pretty low, the frequency of the cosine of the right side of the expression, (f₁−f₂)/2, is usually too low for us to perceive it as a pitch. Instead, we perceive it as a tremolo-pulsating change in the volume of the underlying pitches. The cosine term creates an amplitude-modulated ‘envelope’ for the sine wave (f₁ + f₂)/2, the average of the two pitches. So the dissonances in the tenor and bass parts mainly create a wonderful, shimmering tremolando texture. This is even more dramatic in cathedrals or other echoic spaces, where the reverberation and phase relationships between the parts can add yet more richness and acoustic complexity.

[50-sec clip, Dale Warland Singers, Eric Whitacre, ‘Lux Aurumque’, 1.2MB MP3]

J ames Jordan’s student, Matthew LaPine, offers guidance on how to get singers to properly perceive and deliver these close intervals. He does not talk about beat-frequencies or ‘phantom’ beat-frequency pitches. But Matthew’s discovery that ‘resetting’ what ‘do’ is in your solfege—is a closely-related thing. Our minds associate each solfege syllable and its relation to other syllables/intervals with relative frequency ratios. Resetting where ‘do’ is enables us to better anticipate and accurately produce the dissonances that achieve the proper beat-frequency Terzo Suono.

[50-sec clip, Dale Warland Singers, Eric Whitacre, ‘Lux Aurumque’, 1.2MB MP3]

Dale Warland Singers’ recording of ‘Lux Aurumque’

T he Dale Warland Singers’ recording is my favorite performance of ‘Lux Aurumque’ to-date. Here is another nice performance, on YouTube:

[ King’s College Choir, Cambridge, performing Eric Whitacre’s ‘Lux Aurumque’ ]

T he tight harmonies need to be carefully balanced by a well-trained a cappella choir. Despite this, the descending half-steps tend to be over-shot. The solfege in mm. 13-15 should not be ‘fa-mi-ri, re-re-di-do’. And alternates ‘do-ti...’ and ‘li-la...’ also cause overshoot. Instead, try resetting ‘do’ three times: ‘fa-mi/do-ti, do-do-ti-do’. In other words, treat these as two entirely separate descending chromatic phrases.

C ool. It works. Not just with this piece but with many others. LaPine recommends recasting your solfege with ‘erosive’ resets whenever you are overshooting or whenever you are missing the proper beat-frequency implied pitch. Doing this isn’t rewriting the composition or arguing with the composer regarding what the key signature or mode really are. The whole point is simply to trick your mind and voice into doing the right thing. He says to specifically avoid solfege syllables that would, in your mind, denote a cadence.

D issonances are especially poignant and richly colored when it’s you who’s singing them; they are never as intense for members of the audience. When singing difficult chords with critical dissonances, I like it when singers face each other: that way the phasing of the dissonant notes (the beat-frequency) is even more obvious, easier to hear, and more intensely beautiful.

T he whole phenomenon is, for me, very much like experiencing the music of Alvin Lucier, who also has methodically explored beat-frequency and the physics of sonic-space phasing in his compositions and installation pieces.

If you like, you can click on the screen-shot below and download an Excel spreadsheet where I’ve calculated m2 and M2 combinations in the relevant vocal registers.

Spreadsheet calculating implied pitches of beat-frequencies for major and minor seconds, tenor/alto/soprano

T he Bach chorale-like longer ‘note-against-note’ and shorter ‘figurated’ dissonances with binary divisions of the beat—these, too, are much to be admired in Whitacre’s writing. These interesting dissonances; the graceful, fluent motion from one chord to the next revealing the notes that successive chords have in common; the enriching harmonies with 7ths and 9ths … all without any sacrifice of melodic logic.

L ux Aurumque’ was originally commissioned by the Master Chorale of Tampa Bay, and first arranged for men’s voices for the Gay Men’s Chorus of Los Angeles.

jwilzewske

Saturday, January 3, 2009

The Physical Properties of Sound Itself: Whitacre & Choral Dissonance

No comments:

Post a Comment